How To Find Frequency Of A Wavelength

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for AudioLover.com, at no extra cost. Learn more)

**

Introduction

**

Understanding the relationship between wavelength and frequency is crucial in various scientific and technological fields. Whether you're exploring the realms of physics, engineering, or even telecommunication, the ability to find the frequency of a given wavelength is essential. This article will delve into the fundamentals of wavelength and frequency, elucidate their relationship, and provide practical insights into calculating frequency from a given wavelength.

The concept of wavelength and frequency is not only significant in the realm of science but also in everyday experiences. From the colors we perceive to the signals transmitted wirelessly, these phenomena are omnipresent. By unraveling the intricacies of wavelength and frequency, we can gain a deeper understanding of the world around us and harness this knowledge to innovate and solve real-world problems.

In the subsequent sections, we will explore the foundational principles of wavelength and frequency, demystify the mathematical relationship between the two, and elucidate practical methods for calculating frequency from a given wavelength. Additionally, we will showcase the real-world applications of this knowledge, highlighting its relevance in diverse fields such as telecommunications, astronomy, and medical imaging.

By the end of this article, you will not only grasp the theoretical underpinnings of wavelength and frequency but also appreciate their tangible impact on technology and scientific advancements. Let's embark on this journey to unravel the intricacies of wavelength and frequency and discover the profound significance of this fundamental relationship.

Understanding Wavelength and Frequency

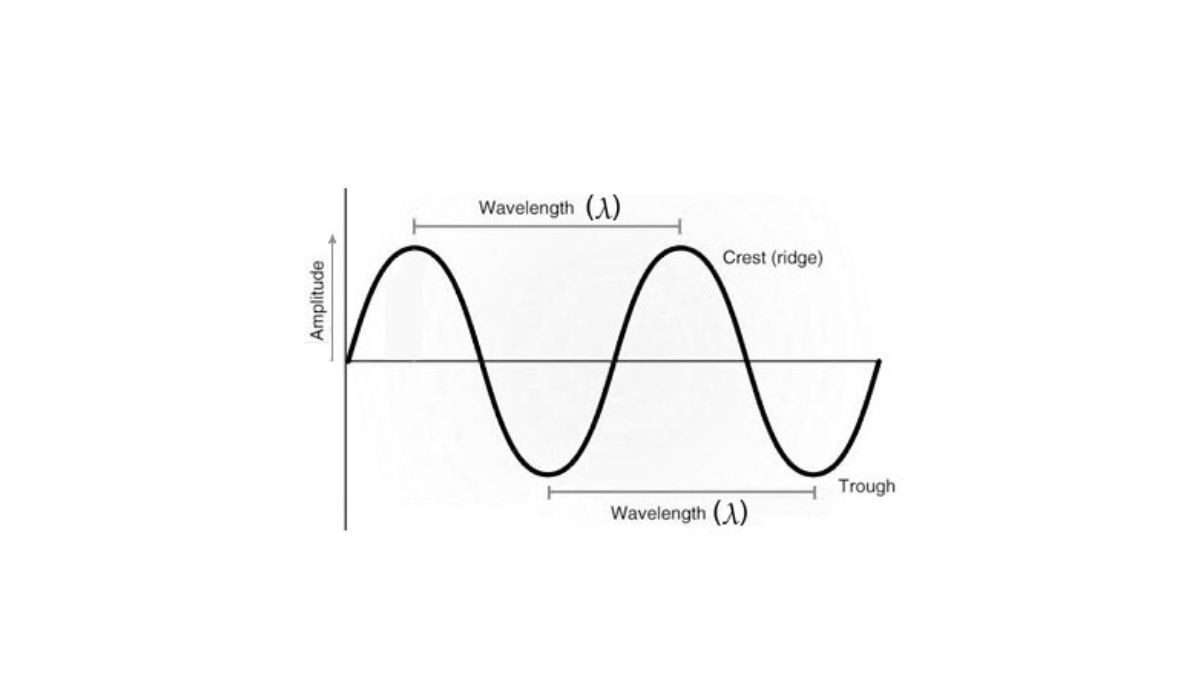
Before delving into the intricacies of finding the frequency of a wavelength, it is imperative to grasp the fundamental concepts of wavelength and frequency. Wavelength refers to the distance between successive crests of a wave, such as an electromagnetic wave or a sound wave. It is commonly denoted by the Greek letter lambda (λ) and is measured in meters (m) or its multiples, such as nanometers (nm) or micrometers (μm).

On the other hand, frequency represents the number of wave cycles that pass through a specific point in a given unit of time. It is typically measured in hertz (Hz), where one hertz corresponds to one cycle per second. In essence, frequency denotes how many wave crests pass a fixed point in a second, indicating the rate of oscillation of the wave.

These two fundamental properties are intrinsically linked, forming the basis of wave behavior across various mediums. In the context of electromagnetic waves, such as light or radio waves, the wavelength determines the type of radiation, while the frequency dictates its energy. In the realm of sound waves, the wavelength influences the pitch of the sound, while the frequency determines its perceived tone.

Understanding the interplay between wavelength and frequency is pivotal in comprehending the behavior of waves across different spectra. Whether it’s the transmission of data through wireless communication, the propagation of light in optical fibers, or the analysis of seismic waves in geophysics, the relationship between wavelength and frequency underpins myriad scientific and technological endeavors.

By grasping the essence of wavelength and frequency, we can unravel the mysteries of wave propagation, decipher the nature of diverse phenomena, and harness this knowledge to innovate and advance various fields. With this foundational understanding in place, we are poised to explore the intricate relationship between wavelength and frequency and its practical implications in the subsequent sections.

The Relationship Between Wavelength and Frequency

The relationship between wavelength and frequency is governed by a fundamental principle: the speed of the wave. This principle is encapsulated in the wave equation, which states that the speed of a wave is equal to the product of its wavelength and frequency. Mathematically, this relationship is expressed as:

speed = wavelength × frequency

This equation underscores the inverse relationship between wavelength and frequency. When the wavelength of a wave increases, its frequency decreases, and vice versa. This phenomenon is particularly evident in the realm of electromagnetic waves, where different types of radiation, such as radio waves, microwaves, and visible light, exhibit distinct wavelengths and frequencies.

For instance, in the electromagnetic spectrum, radio waves have relatively long wavelengths, ranging from meters to kilometers, and correspondingly low frequencies in the kilohertz to megahertz range. In contrast, visible light encompasses a spectrum of shorter wavelengths, spanning from approximately 400 to 700 nanometers, and higher frequencies in the hundreds of terahertz.

Understanding this inverse relationship is pivotal in various applications. In telecommunications, for instance, the allocation of different frequency bands for wireless communication is predicated on optimizing the trade-off between wavelength and frequency. Similarly, in spectroscopy and remote sensing, the distinctive wavelengths and frequencies of electromagnetic radiation enable the identification and analysis of diverse materials and phenomena.

Moreover, in the context of sound waves, the relationship between wavelength and frequency manifests in the pitch and tone of the sound. Longer wavelengths correspond to lower frequencies, resulting in deeper tones, while shorter wavelengths are associated with higher frequencies, yielding sharper pitches. This interplay between wavelength and frequency forms the basis of musical harmony and the auditory experience.

By comprehending the intricate relationship between wavelength and frequency, we can navigate the diverse manifestations of waves, from the transmission of wireless signals to the propagation of light and sound. This foundational understanding sets the stage for delving into the practical methods of calculating frequency from a given wavelength, which we will explore in the subsequent section.

Calculating Frequency from Wavelength

Calculating the frequency from a given wavelength involves employing the fundamental relationship between wavelength, frequency, and the speed of the wave. This relationship is encapsulated in the wave equation, which states that the speed of a wave is equal to the product of its wavelength and frequency. Rearranging this equation allows us to calculate the frequency when the wavelength and speed of the wave are known:

frequency = speed / wavelength

Where:

• frequency represents the number of wave cycles per unit of time, measured in hertz (Hz).
• speed denotes the speed of the wave, typically denoted by the symbol c for electromagnetic waves (e.g., speed of light in a vacuum) or v for other types of waves, measured in meters per second (m/s).
• wavelength signifies the distance between successive wave crests, measured in meters (m).

By utilizing this formula, we can determine the frequency of a wave when its wavelength and speed are known. For instance, in the context of light waves traveling in a vacuum, the speed of light (c) is approximately 3.00 x 10^8 meters per second. If the wavelength of the light is 500 nanometers (5.00 x 10^-7 meters), we can calculate the frequency as follows:

frequency = (3.00 x 10^8 m/s) / (5.00 x 10^-7 m) = 6.00 x 10^14 Hz

This calculation demonstrates how the relationship between wavelength, frequency, and the speed of the wave enables us to determine the frequency from the given wavelength. Whether it’s analyzing the characteristics of electromagnetic radiation, discerning the properties of sound waves, or optimizing the performance of communication systems, the ability to calculate frequency from wavelength is indispensable in diverse scientific and technological domains.

Moreover, this fundamental relationship serves as a cornerstone for myriad applications, from designing wireless networks and optimizing signal transmission to elucidating the behavior of waves in different mediums. By harnessing this knowledge, scientists, engineers, and innovators can unravel the complexities of wave phenomena and leverage them to advance a myriad of fields, from telecommunications and astronomy to medical diagnostics and beyond.

As we delve into the practical applications of finding frequency from wavelength, it becomes evident that this foundational understanding forms the bedrock of numerous technological innovations and scientific discoveries, underscoring its profound significance in our quest to comprehend and harness the behavior of waves across diverse spectra.

Practical Applications of Finding Frequency from Wavelength

The ability to find the frequency from a given wavelength holds immense practical significance across a myriad of scientific and technological domains. One notable application lies in the realm of telecommunications, where the allocation of frequency bands for wireless communication is predicated on optimizing the trade-off between wavelength and frequency. By precisely determining the frequency corresponding to a specific wavelength, engineers can design and deploy wireless networks that facilitate seamless data transmission and connectivity.

Another compelling application resides in the field of spectroscopy, where the analysis of electromagnetic radiation’s wavelength and frequency enables the identification and characterization of diverse materials and compounds. By leveraging the unique spectral signatures of different substances, scientists can employ spectroscopic techniques to discern the composition, structure, and properties of substances, ranging from environmental samples to biological molecules.

Furthermore, in the domain of medical imaging, the relationship between wavelength and frequency underpins various diagnostic modalities, such as ultrasound and magnetic resonance imaging (MRI). By exploiting the distinct wavelengths and frequencies of sound waves and radio waves, medical practitioners can visualize internal anatomical structures, detect abnormalities, and diagnose medical conditions with precision and accuracy.

Moreover, in the field of astronomy, the determination of frequency from the wavelength of celestial radiation is instrumental in unraveling the mysteries of the cosmos. Whether it’s analyzing the spectral lines emitted by distant stars or probing the cosmic microwave background, astronomers rely on the principles of wavelength and frequency to glean insights into the composition, temperature, and dynamics of celestial objects and phenomena.

Additionally, the interplay between wavelength and frequency finds application in environmental monitoring and geophysics, where the analysis of seismic waves and electromagnetic signals aids in understanding geological processes, detecting natural hazards, and surveying subsurface structures. By discerning the wavelengths and frequencies of these signals, scientists can infer valuable information about the Earth’s composition and dynamics.

By elucidating the practical applications of finding frequency from wavelength, it becomes evident that this foundational knowledge permeates diverse fields, from telecommunications and medicine to astronomy and geophysics. The ability to unravel the intricacies of wave behavior and leverage this understanding to innovate and solve real-world challenges underscores the profound significance of this fundamental relationship in advancing science, technology, and our collective understanding of the natural world.

Conclusion

In conclusion, the relationship between wavelength and frequency constitutes a fundamental tenet that underpins the behavior of waves across diverse spectra, from electromagnetic radiation to sound waves. By unraveling the intricacies of this relationship and delving into the practical methods of finding frequency from a given wavelength, we gain a profound understanding of the profound impact of this fundamental principle on myriad scientific and technological endeavors.

From the realms of telecommunications and spectroscopy to medical imaging, astronomy, and geophysics, the ability to determine the frequency corresponding to a specific wavelength enables scientists, engineers, and innovators to propel technological innovations, unravel the mysteries of the cosmos, and solve real-world challenges. Whether it’s optimizing wireless communication networks, characterizing materials through spectroscopic analysis, visualizing internal anatomical structures, or probing the depths of the Earth, the foundational knowledge of wavelength and frequency serves as a gateway to transformative advancements.

Moreover, this understanding fosters a profound appreciation for the ubiquitous nature of wave phenomena in our everyday experiences, from the colors we perceive to the signals that enable global connectivity. By comprehending the interplay between wavelength and frequency, we not only unravel the mysteries of wave propagation but also harness this knowledge to innovate, advance scientific frontiers, and enhance our collective understanding of the natural world.

As we continue to explore the frontiers of science and technology, the foundational principles of wavelength and frequency will undoubtedly remain pivotal, serving as guiding beacons in our quest to decipher the complexities of wave behavior and leverage this understanding to drive progress and innovation. By embracing the profound significance of this fundamental relationship, we embark on a journey of discovery, innovation, and transformation, poised to unravel the mysteries of the universe and harness the power of waves to shape a brighter future for humanity.