How To Calculate Fundamental Frequency

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for AudioLover.com, at no extra cost. Learn more)

Introduction

Understanding the Basics of Fundamental Frequency

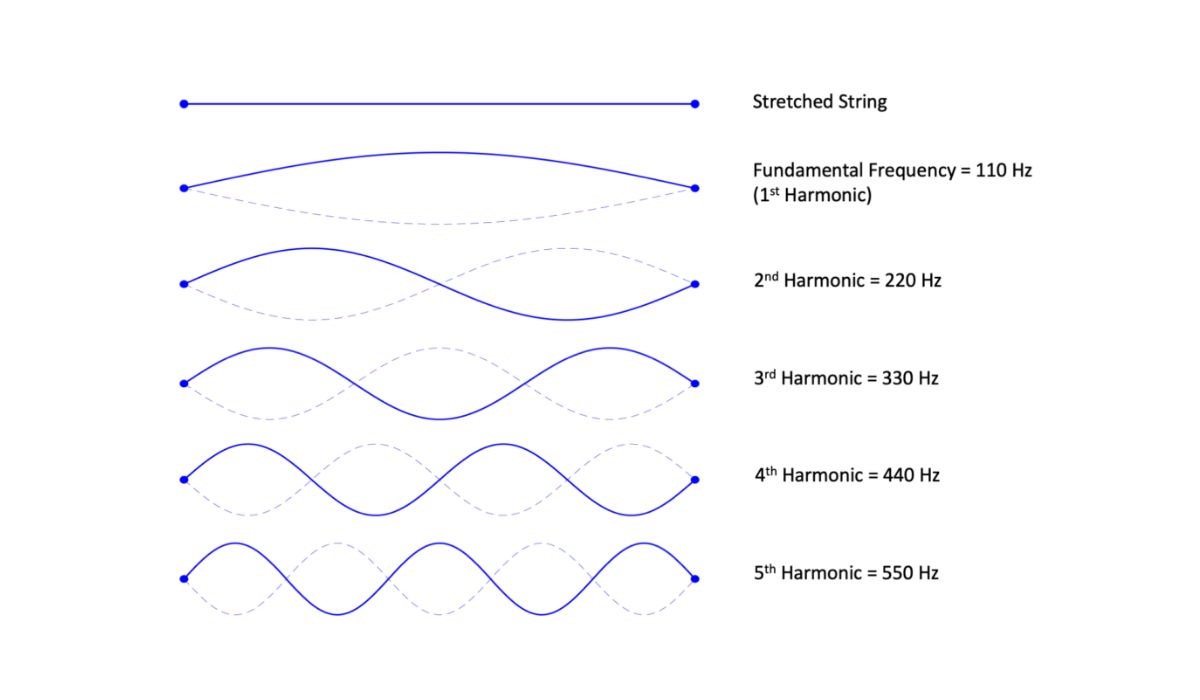
Understanding the fundamental frequency is crucial in various fields, including music, acoustics, and signal processing. The fundamental frequency, often referred to as the first harmonic, is the lowest frequency at which a system vibrates. In simpler terms, it represents the base pitch of a sound or the fundamental tone of a musical note. This fundamental frequency forms the basis for all other frequencies present in a complex waveform, making it a fundamental concept in the study of waveforms and sound.

The determination of the fundamental frequency is essential in music theory, as it dictates the perceived pitch of a musical note. In speech and language analysis, the fundamental frequency plays a vital role in understanding intonation patterns and vocal characteristics. Moreover, in the field of signal processing, identifying the fundamental frequency is crucial for tasks such as pitch detection, speech recognition, and audio coding.

In this comprehensive guide, we will delve into the intricacies of fundamental frequency, exploring the methods for calculating it and its significance in various applications. By the end of this article, you will have a clear understanding of how fundamental frequency is calculated and its relevance in different domains. Let's embark on this enlightening journey to unravel the mysteries of fundamental frequency and its profound impact on the world of sound and waveforms.

Understanding Fundamental Frequency

Exploring the Essence of Fundamental Frequency

Before delving into the intricacies of calculating the fundamental frequency, it’s imperative to grasp the underlying principles of this fundamental concept. The fundamental frequency is the lowest frequency produced by a vibrating object or sound wave, representing the primary building block of a complex waveform. In the context of music, the fundamental frequency determines the perceived pitch of a musical note, laying the foundation for the entire harmonic series associated with that note.

When a sound wave is generated, it consists of multiple frequencies, each with varying amplitudes and phases. The lowest of these frequencies is the fundamental frequency, which gives the sound its characteristic pitch. For instance, in a musical instrument such as a guitar, the fundamental frequency corresponds to the pitch of the open string, serving as the reference point for the harmonics produced when the string is plucked or strummed.

Understanding the fundamental frequency is pivotal in the analysis of complex waveforms, as it provides insights into the tonal quality and timbre of a sound. In speech and language, variations in fundamental frequency contribute to the nuances of intonation and convey emotional cues, playing a crucial role in effective communication and expression.

Furthermore, in the realm of signal processing, the fundamental frequency serves as a fundamental parameter for tasks such as pitch detection, audio synthesis, and sound analysis. By isolating and analyzing the fundamental frequency component of a waveform, researchers and practitioners can extract valuable information about the source of the sound and make informed decisions in various applications.

By comprehending the essence of fundamental frequency, we gain a deeper appreciation for the role it plays in shaping the auditory world around us. In the subsequent sections, we will explore the methodologies for calculating the fundamental frequency, shedding light on the techniques used to extract this fundamental component from complex waveforms.

Determining the Period of the Waveform

Unraveling the Temporal Characteristics of Waveforms

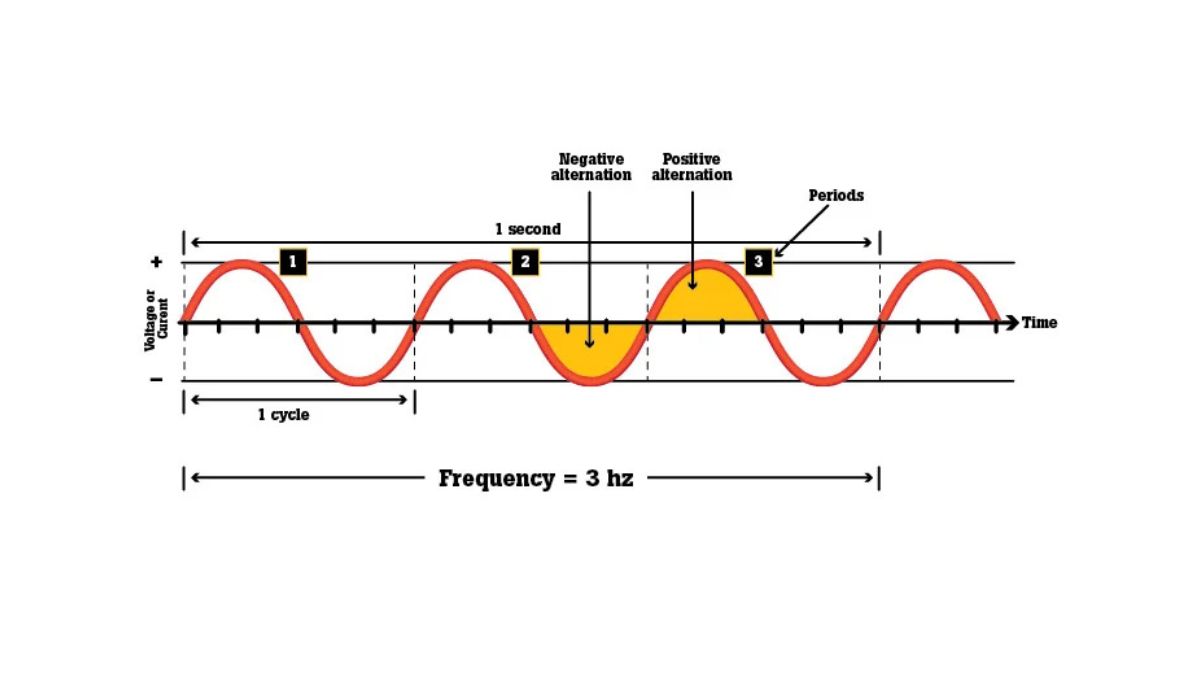
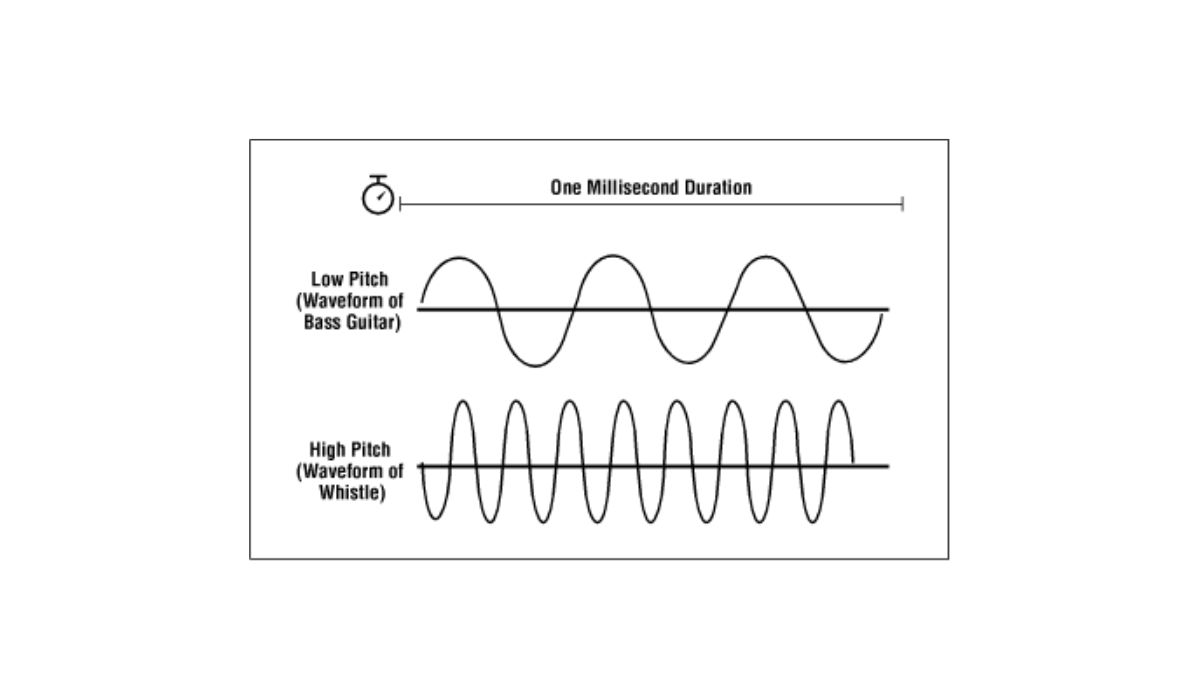
Before delving into the intricacies of calculating the fundamental frequency, it’s essential to understand the concept of the waveform period. The period of a waveform refers to the time it takes for one complete cycle of the waveform to occur. In the context of sound waves, the period represents the duration of a single oscillation, which directly influences the frequency and pitch of the sound.

To determine the period of a waveform, one must identify the time interval between two consecutive points that exhibit the same phase and direction within the waveform. In a sine wave, for instance, the period can be measured as the time taken for the waveform to complete one full cycle, starting from a specific point on the waveform and ending at the same point in the subsequent cycle.

The period of a waveform is inversely proportional to its frequency, as higher frequencies correspond to shorter periods, indicating a higher rate of oscillation within a given time frame. In practical terms, the period of a waveform directly influences the perceived pitch of a sound, with shorter periods corresponding to higher pitches and longer periods yielding lower pitches.

Understanding the period of a waveform is pivotal in the context of fundamental frequency calculation, as it forms the basis for determining the frequency of the waveform. By accurately measuring the period of a waveform, one can derive the fundamental frequency, which serves as a fundamental parameter in various fields, including music, acoustics, and signal processing.

As we delve deeper into the methodologies for calculating the fundamental frequency, the concept of waveform period will emerge as a crucial element in extracting the fundamental component from complex waveforms. By unraveling the temporal characteristics of waveforms and understanding their periodic nature, we pave the way for accurate and insightful fundamental frequency calculations, contributing to advancements in diverse domains reliant on sound and waveform analysis.

Calculating Fundamental Frequency Using Period

Deriving Fundamental Frequency from Waveform Period

One of the fundamental methods for calculating the fundamental frequency of a waveform involves leveraging the concept of waveform period. The fundamental frequency, denoted as f₀, is reciprocally related to the period of the waveform, as expressed by the equation:

f₀ = 1 / T

Where f₀ represents the fundamental frequency, and T denotes the period of the waveform. By measuring the period of a waveform, one can directly compute the fundamental frequency, providing valuable insights into the pitch and tonal characteristics of the sound.

The process of calculating the fundamental frequency using the waveform period entails identifying the time interval for one complete cycle of the waveform, as discussed in the previous section. Once the period is determined, the reciprocal of the period yields the fundamental frequency, representing the base pitch of the sound wave.

For instance, if the period of a waveform is measured to be 0.02 seconds, the fundamental frequency can be calculated using the formula f₀ = 1 / 0.02, resulting in a fundamental frequency of 50 Hz. This indicates that the sound wave completes 50 cycles per second, influencing the perceived pitch and contributing to the overall auditory experience.

Calculating the fundamental frequency using the waveform period serves as a fundamental technique in various applications, including music theory, acoustics, and signal processing. By accurately determining the period of a waveform and applying the fundamental frequency formula, researchers and practitioners can gain valuable insights into the tonal quality, pitch, and harmonic characteristics of complex waveforms.

As we explore the methodologies for calculating the fundamental frequency, the utilization of waveform period as a foundational parameter underscores its significance in unraveling the spectral composition of sound waves and extracting essential frequency components. By harnessing the relationship between waveform period and fundamental frequency, we pave the way for a deeper understanding of the auditory world and the intricate patterns that define the essence of sound.

Calculating Fundamental Frequency Using Waveform Samples

Utilizing Signal Processing Techniques for Fundamental Frequency Analysis

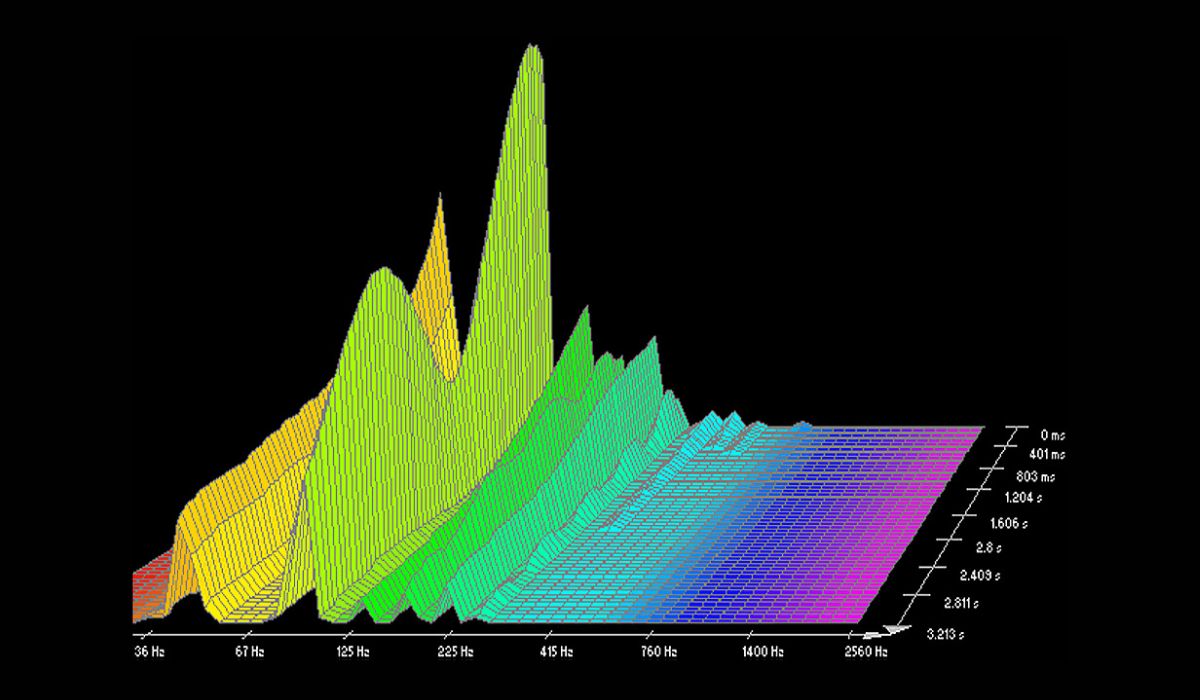
Another prominent approach for calculating the fundamental frequency of a waveform involves leveraging signal processing techniques and analyzing waveform samples. In this method, a series of discrete samples from the waveform are processed to extract the fundamental frequency, offering a comprehensive understanding of the spectral components and harmonic structure of the sound.

Signal processing algorithms, such as the Fast Fourier Transform (FFT), play a pivotal role in analyzing waveform samples to identify the dominant frequency components and determine the fundamental frequency. By converting the time-domain waveform into its frequency-domain representation, signal processing techniques enable the decomposition of complex waveforms into their constituent frequencies, facilitating the extraction of the fundamental frequency.

The process of calculating the fundamental frequency using waveform samples involves the following steps:

• Sampling the Waveform: The continuous waveform is sampled at regular intervals to obtain discrete data points, which are subsequently processed using signal processing algorithms.
• FFT Analysis: The sampled waveform undergoes FFT analysis to transform it from the time domain to the frequency domain, revealing the amplitude and frequency components present in the signal.
• Fundamental Frequency Detection: By analyzing the FFT results, the dominant frequency component corresponding to the fundamental frequency is identified, providing a precise measure of the base pitch of the sound.

By employing signal processing techniques and analyzing waveform samples, researchers and practitioners can gain valuable insights into the fundamental frequency of complex waveforms, enabling detailed spectral analysis and harmonic characterization of the sound. This method is particularly beneficial in scenarios where precise frequency measurements and in-depth waveform analysis are essential, such as in music production, speech analysis, and acoustic research.

Furthermore, the utilization of waveform samples and signal processing techniques allows for real-time fundamental frequency estimation, making it a valuable tool in applications such as pitch detection, audio synthesis, and sound recognition systems. The ability to accurately calculate the fundamental frequency from waveform samples contributes to advancements in diverse fields, fostering innovation and enhancing our understanding of the intricate nature of sound and waveforms.

As we delve into the methodologies for fundamental frequency calculation, the utilization of waveform samples and signal processing techniques underscores the dynamic intersection of technology and sound analysis, paving the way for comprehensive and nuanced insights into the fundamental components of complex waveforms.

Conclusion

Unveiling the Harmonic Foundations of Sound

Throughout this enlightening exploration of fundamental frequency calculation, we have delved into the fundamental principles that underpin the spectral composition of sound waves and the methodologies for extracting the fundamental frequency from complex waveforms. The fundamental frequency, serving as the cornerstone of pitch perception and harmonic analysis, plays a pivotal role in shaping the auditory landscape across diverse domains, including music, acoustics, and signal processing.

By understanding the temporal and spectral characteristics of waveforms, we gain valuable insights into the tonal quality, pitch, and harmonic structure of sound, paving the way for precise fundamental frequency calculations. Whether through the measurement of waveform periods or the utilization of signal processing techniques to analyze waveform samples, the methodologies presented in this guide offer comprehensive approaches for deriving the fundamental frequency, empowering researchers and practitioners to unravel the intricate patterns that define the essence of sound.

The ability to accurately calculate the fundamental frequency contributes to advancements in music theory, speech analysis, audio production, and a myriad of other applications reliant on sound and waveform analysis. From the resonant tones of musical instruments to the nuances of human speech, the fundamental frequency serves as a fundamental parameter in understanding and interpreting the auditory world.

As technology continues to evolve, the intersection of signal processing algorithms, waveform analysis, and fundamental frequency calculation opens new frontiers in sound research and innovation. Real-time fundamental frequency estimation, precise pitch detection, and advanced audio synthesis techniques are just a few examples of the transformative applications that stem from a deep understanding of fundamental frequency principles.

In conclusion, the profound impact of fundamental frequency calculation resonates across disciplines, shaping the way we perceive and interact with sound. By unraveling the harmonic foundations of sound through meticulous analysis and calculation, we embark on a journey of discovery, unlocking the mysteries of sound waves and their fundamental components, ultimately enriching our auditory experiences and advancing the frontiers of sound technology.