How To Do Intervals In Music Theory

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for AudioLover.com, at no extra cost. Learn more)

Introduction

Welcome to the fascinating world of music theory! If you have ever wondered how melodies are crafted, harmonies are formed, or chords are structured, then understanding intervals is a fundamental aspect of diving deeper into the realm of music theory.

Intervals are the building blocks of music. They are defined as the distance between two pitches or notes. Just like the alphabet has letters that make up words, intervals are the language that musicians use to communicate and create beautiful compositions. Whether you are a beginner seeking to understand the basics or an experienced musician looking to expand your knowledge, this article will guide you through the concept of intervals in music theory.

Understanding intervals is crucial for various aspects of music, including melody writing, chord progressions, harmonizing melodies, transposing music, and improvisation. It allows you to analyze and appreciate the nuances of musical compositions, enabling you to express yourself more effectively as a musician and composer.

In this article, we will explore the different types of intervals, how they are built, the notations used to represent them, the concept of interval inversions, and how intervals can be utilized in composition. By the end, you will have a solid understanding of intervals and their significance in music theory.

So, let’s embark on this musical journey as we unravel the mysteries behind intervals in music theory and discover the enchanting world that lies within!

Understanding Intervals in Music Theory

Intervals are the building blocks of music, and understanding them is crucial for interpreting and creating music. In music theory, intervals are defined as the distance between two pitches or notes. They provide a framework for understanding the relationships between different notes and their role in creating melodies, harmonies, and chords.

Intervals are classified based on two factors: the distance in pitch between the two notes and their position within the musical scale. The distance is measured in steps, with each step representing a specific interval. The position within the scale determines whether the interval is major, minor, perfect, diminished, or augmented.

For example, let’s consider the C major scale: C, D, E, F, G, A, B, C. If we take two consecutive notes, such as C and D, we have created an interval. This particular interval is called a major 2nd, as it consists of two steps from the starting note.

Intervals can also be classified by their size. The smallest interval in Western music is the half step, which is equivalent to one key on the piano. The largest interval is the octave, which spans the distance of eight notes.

Understanding intervals allows musicians to analyze and interpret melodies, harmonize vocals or instruments, and transpose music into different keys. It also plays a significant role in composition and improvisation, as musicians can utilize intervals to create tension, resolution, and emotional depth in their music.

By grasping the concept of intervals, you can unlock a new level of musicality and enhance your ability to express yourself as a musician. With this foundation in place, let’s explore the different types of intervals and how they are constructed in music theory.

Types of Intervals

In music theory, intervals are classified into different types based on their size and quality. This classification helps us understand the characteristics and relationships between notes within a musical composition. Let’s explore the main types of intervals:

1. Perfect Intervals: Perfect intervals are considered the most consonant and stable intervals. In Western music, the perfect intervals include the unison (P1), fourth (P4), fifth (P5), and octave (P8). These intervals have a pure, harmonious sound and are commonly used in melodies and harmonies.
2. Major Intervals: Major intervals have a larger size compared to perfect intervals. They convey a brighter and more uplifting quality. The major intervals include the major second (M2), major third (M3), major sixth (M6), and major seventh (M7).
3. Minor Intervals: Minor intervals are slightly smaller than major intervals and have a darker, more melancholic sound. The minor intervals include the minor second (m2), minor third (m3), minor sixth (m6), and minor seventh (m7).
4. Augmented Intervals: Augmented intervals are larger than major or perfect intervals. They have a more dissonant and tense sound. For example, the augmented second (A2) is equivalent to a major third, but with a sharper or higher pitch.
5. Diminished Intervals: Diminished intervals are smaller than perfect or minor intervals. They create a dissonant and unstable sound. For instance, the diminished fifth (d5) is a tritone, which is known for its unresolved, “devil in music” quality.

Understanding the different types of intervals and their characteristics is essential for analyzing and interpreting melodies, harmonies, and chords. It enables musicians to build and structure musical phrases, create tension and resolution, and establish the overall mood and emotion of a composition.

Now that we have a basic understanding of the various types of intervals, let’s dive deeper into how intervals are constructed in music theory.

Building Intervals

In music theory, intervals are built by measuring the distance between two pitches or notes. The process of constructing intervals involves understanding the steps or half steps between the notes and applying the interval formula.

The interval formula determines the number of steps required to reach the desired interval. It is based on the concept of the major scale, which serves as the foundation for interval construction. Each note in the major scale represents a specific degree or step, which helps determine the distance between two notes.

Let’s take the C major scale as an example: C, D, E, F, G, A, B, C. To build an interval, count the number of steps from the starting note to the ending note, including both the starting and ending notes themselves.

For instance, to construct a major second interval above the note C, count two steps up the major scale. This leads us to the note D, resulting in a major second interval.

Similarly, to create a minor third interval above the note A, count three steps up the major scale. This brings us to the note C, resulting in a minor third interval.

It’s important to note that not all intervals are found within the major scale. Some intervals, such as the perfect intervals (unison, fourth, fifth, octave), have their own unique formulas.

Additionally, intervals can be inverted by reversing the order of the notes. For example, if we have a major sixth interval from C to A, the inversion of this interval would be a minor third (A to C).

Understanding how to construct intervals allows musicians to accurately identify and communicate the distance between notes, enabling them to transpose music to different keys, create harmonies, and develop melodic ideas.

Now that we have covered the process of building intervals, let’s dive into the techniques used to notate them in music theory.

Notating Intervals

In music theory, intervals are notated using a combination of numbers and symbols. This notation system provides a standardized way to represent the distance between two pitches or notes.

Intervals are typically notated using two numbers: the quality and the number. The quality refers to whether the interval is perfect, major, minor, augmented, or diminished, while the number represents the distance between the two notes, measured in steps.

For example, a major third interval is notated as M3, where “M” denotes major and “3” represents the distance of three steps. A perfect fifth interval is notated as P5, with “P” indicating perfect and “5” indicating five steps.

Here is a summary of the symbols used to notate intervals:

• “P” – Perfect
• “M” – Major
• “m” – Minor
• “A” – Augmented
• “d” – Diminished

The number associated with each interval represents the number of steps or degrees between the two notes. For example, a major second has a distance of two steps, and a minor third has a distance of three steps.

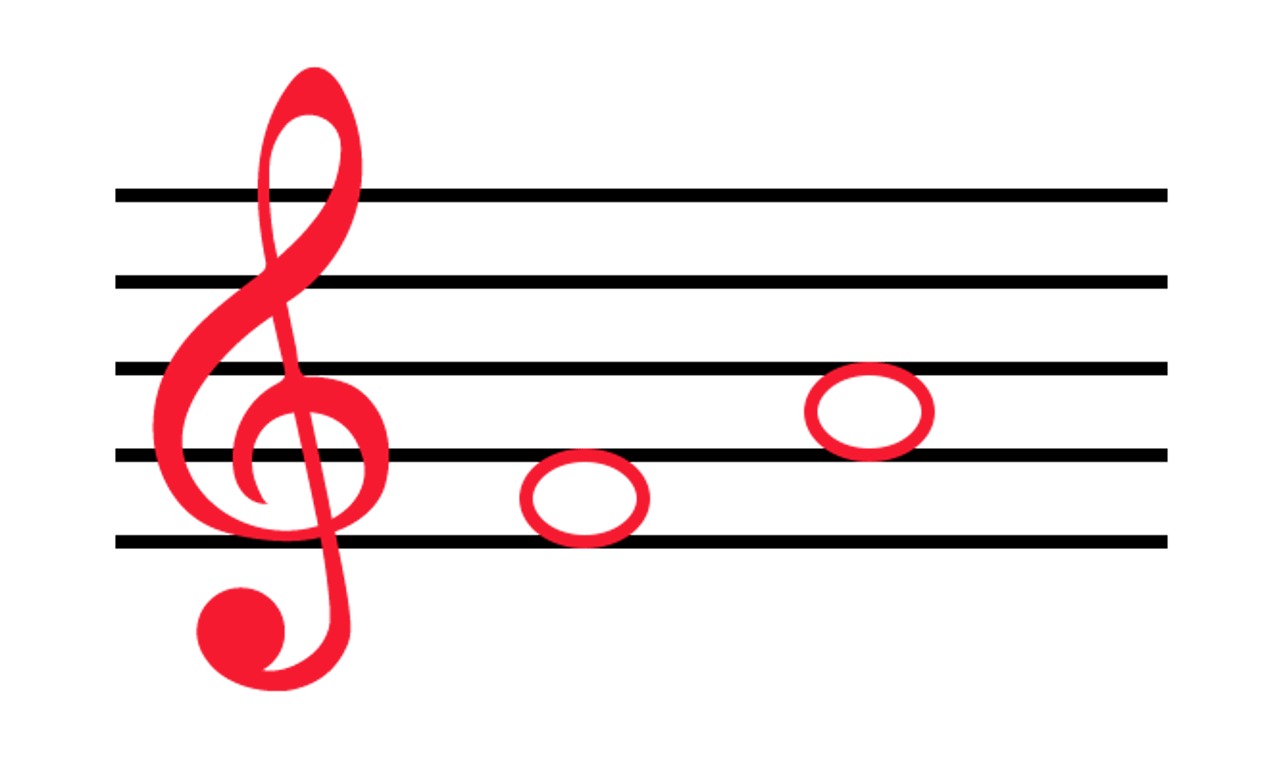
In addition to the above symbols, intervals can also be notated using staff notation, which represents the specific pitches on the musical staff. This notation provides a visual representation of the interval on the musical staff, allowing musicians to easily identify and read the intervals.

For example, the interval from the C note to the E note can be notated as a major third using staff notation. The C and E notes will be placed on the corresponding lines or spaces on the staff, illustrating the distance between them.

Understanding how to notate intervals is crucial for reading sheet music, analyzing compositions, and communicating musical ideas with other musicians. It provides a universal language for musicians to understand and interpret intervals accurately.

Now that we have covered how to notate intervals, let’s explore the concept of interval inversions and their significance in music theory.

Interval Inversions

In music theory, interval inversions refer to reversing the order of the notes in an interval. This process results in a new interval that is complementary to the original interval.

To find the inversion of an interval, you need to calculate the distance between the lower note and the nearest octave of the higher note. The resulting distance will determine the quality and number of the inverted interval.

For example, if we have a major third interval from C to E, the inversion of this interval would be a minor sixth (E to C). The distance between the lower note (E) and the nearest octave of the higher note (C) is six steps, resulting in a minor sixth interval.

Let’s look at a few more examples of interval inversions:

• A perfect fourth (C to F) is inverted to a perfect fifth (F to C).
• A major seventh (C to B) is inverted to a minor second (B to C).
• A minor third (C to E♭) is inverted to a major sixth (E♭ to C).

Interval inversions are essential for understanding harmonic relationships and creating harmonizations. They allow musicians to expand their melodic and harmonic possibilities by exploring different intervals and their inversions.

In addition to understanding inversion, it is important to note that certain intervals are their own inversions. These intervals include the unison (P1) and the octave (P8). In other words, when an interval is inverted, it remains the same.

Interval inversions provide a fresh perspective on melodies and harmonies, allowing musicians to create unique and interesting musical ideas. It is a concept that composers, arrangers, and improvisers frequently utilize to add depth and variation to their compositions.

Now that we have explored interval inversions, let’s move on to how intervals can be effectively used in composition.

Using Intervals in Composition

Intervals play a vital role in composition, allowing musicians to create melodies and harmonies that evoke specific emotions and enhance the overall musical experience. By understanding how intervals work and how they interact with one another, composers can effectively craft melodies and harmonies that are memorable and captivating.

One approach to using intervals in composition is by considering their inherent qualities and emotional connotations. For example, perfect intervals often create a sense of stability and consonance, making them ideal for establishing strong harmonies and defining the tonal center of a piece. On the other hand, dissonant intervals, like the tritone, can be utilized to create tension and add color to a composition.

Using intervals in melody writing allows composers to create memorable and distinct musical phrases. By incorporating intervals of different sizes and qualities, composers can add variety and interest to their melodies. A mixture of small and large intervals can create melodic leaps and steps, adding dynamics and movement to the composition.

Harmonically, intervals are the foundation of chords and chord progressions. Composers often use specific intervals to build chords that convey different moods and emotions. For example, major chords are constructed using a major third and a perfect fifth, giving them a bright and uplifting quality. Minor chords, on the other hand, are built with a minor third and a perfect fifth, resulting in a more melancholic or introspective sound.

Intervals can also be used in composition to create harmonizations and counterpoint. By layering different melodies or voices, composers can explore the interplay between intervals, creating rich textures and harmonies. Understanding and manipulating intervals allow composers to create harmonic progressions and voice arrangements that are expressive and engaging.

Furthermore, composers can use intervals to modulate or transpose a piece of music into a different key. By shifting the starting pitches of the intervals, the entire composition can be transformed, offering variations and new perspectives to the listener.

Ultimately, the use of intervals in composition is a powerful tool for expressing emotions, developing melodic ideas, constructing harmonies, and creating unique musical experiences. By harnessing the potential of intervals, composers can craft beautiful and impactful compositions that resonate with their audience.

Now that we have explored the various aspects of using intervals in composition, let’s wrap up our discussion.

Conclusion

Intervals are the essence of music theory, serving as the foundation for melodies, harmonies, chords, and compositions. By understanding and utilizing intervals, musicians and composers can enhance their understanding of music, effectively communicate their artistic intentions, and create captivating musical experiences.

In this article, we have explored the fundamental concepts of intervals in music theory. We began by introducing the significance of intervals and how they are the building blocks of music. Then, we delved into the different types of intervals, including perfect, major, minor, augmented, and diminished intervals, each with its unique qualities and emotional connotations.

We further explored the process of building intervals and how they are notated, both in numerical and staff notation. Understanding how intervals are constructed and represented allows musicians to accurately communicate and interpret musical ideas.

We also discussed the concept of interval inversions, which involves reversing the order of notes to create complimentary intervals. Interval inversions open up new possibilities for melodic variation, harmonic explorations, and creating musical tension and resolution.

Lastly, we explored the practical application of intervals in composition. We discussed how intervals can be effectively used in melody writing, harmonization, chord progressions, counterpoint, modulation, and transposition. By harnessing the power of intervals, composers can express emotions, create memorable melodies, and craft harmonies that resonate with their listeners.

Understanding intervals in music theory provides musicians with a deeper appreciation and comprehension of the language of music. It empowers them to analyze, interpret, and express musical ideas with precision and artistry.

As you continue your journey in music theory, remember that intervals are not merely academic concepts; they are the means through which music comes alive and touches the souls of listeners. So, embrace the enchanting world of intervals and let your musical imagination soar!